What is a trojan body?

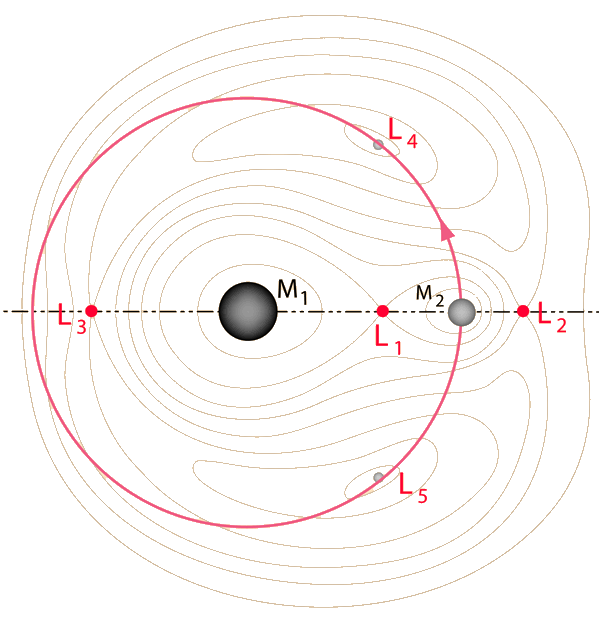Around one hundred years after the publication of the basics of gravitational forces in Principia Mathematica (1687) by Sir Isaac Newton, Joseph-Louis Lagrange studied its implications in the three-body problem. Five stable points in the gravitational field of two massive bodies arose from his calculations. We now call these regions as Lagrange points L1, L2, L3, L4, and L5. The first three are located in the line connecting the two massive bodies, while L4 and L5 form two equilateral triangles with them (see diagram on the right). The latter ones are known to be very stable, so that additional bodies can get trapped in these regions and keep librating in this gravity wells. This "small" bodies co-orbiting with a larger body (usually a planet) around a more massive object (usually a star) are called Trojans. The origin of the name is obvious when we remember the history of the Troy war described in the Iliad of Homero.

Trojans in our Solar System

Examples of this type of configurations are found in our Solar System. The most well-known Trojans are found in Jupiter's L4 and L5 points, but other planets like Venus, Mars, Neptune, Uranus, and our own Earth also host Trojan bodies (see below). Jupiter has the largest amount of trojans known in the Solar System, hosting more than 150000 bodies larger than 1 km in both Lagrangian points L4 and L5, with 624 Hektor being the largest trojan found so far and having a diameter of around 203 km. A much smaller number of trojans has been found in the orbits of Mars and Neptune. In the first case, seven bodies have been confirmed so far, all of them having sizes around 1km or smaller, and most of them librating around L5, i.e., trailing the planet. In the case of Neptune, 12 trojans have been detected so far according to the Minor Planet Center. Their sizes range between 50-200 km

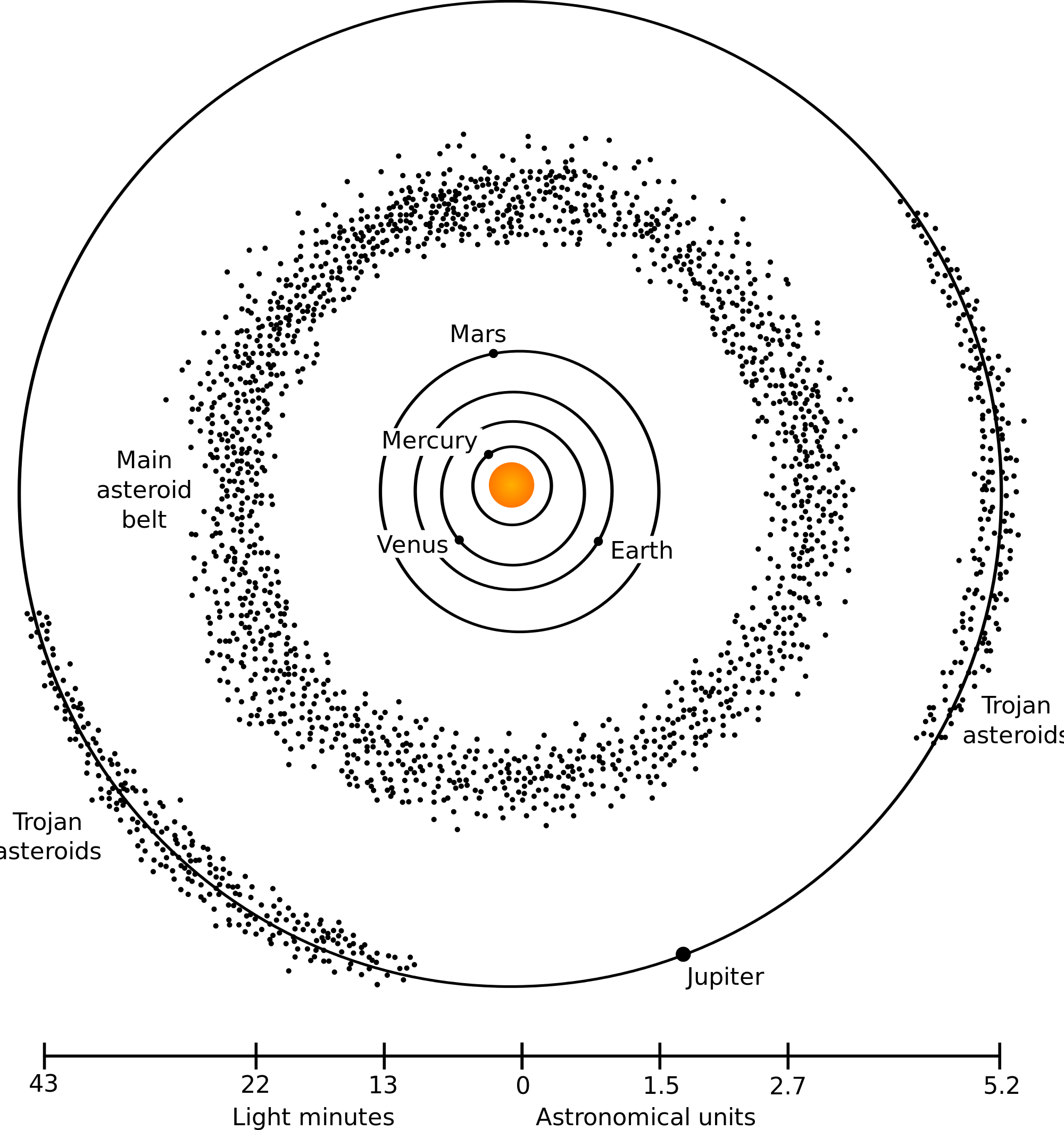
Interestingly, not only planets but also moons can have their own Trojans, as for instance the case of Telesto and Calipso, located at L4 and L5 points of the Tethys Saturnian moon.

The Earth trojans

In 2011, Connors et al. (2011) detected the first trojan body co-orbiting in Earth's orbit, 2010 TK7. This body has an estimated average diameter of around 300 m. Its orbit around L4 is highly inclined (i=20.9º) and eccentric (e=0.191). Interestingly, Connors et al. (2011) derived that this body jumped from L5 to L4 around 2400 years ago, probably due to its large eccentricity. This L4-L5 transition has also been found in some Jupiter trojans (e.g., Tsiganis et al., 2000).

More recently, other objects have been found to co-orbit with our planet and currently, up to 17 objects are known to inhabit our orbit. However, most of them are located in rather unstable orbits and so will scape from the co-orbital motion in the near future.

The discovery of these co-orbital bodies to the Earth is also interesting because they share the irradiation properties of the Earth. Consequently, depending on their composition, the time that thay have been co-orbiting the Earth in an stable orbit and their libration properties, these bodies could be good candidates for fly-by exploratory mission. While no mission has yet been planned to a trojan asteroid, sending a spacecraft to the LAgrangian points of the Earth could provide many information about the population of bodies in that regiions. It is important to note that L4 and L5 are not visible from the Earth since their visibility just occurs during day time.

Why do we care about trojans in extrasolar systems?

It is clear that trojan bodies are outgrowths of the planet formation process and the subsequent planet migration that we now know is essential to explain the current diversity of the known planet population. Thus, they are tracers of these processes and they must exist in any other outer planetary systems. Detecting them will bring up hidden pieces of the puzzle of planet formation.

Relevant conclusions from the literature

Formation:
  • In situ formation (Laughlin et al. 2002): "Recent high-resolution numerical simulations by Balmforth & Korycansky (2001) have shown that the large-scale vortical flow enveloping the horseshoe region nucleates additional smaller vortices, which may spur further formation of planetary cores."

Migration:
  • "Once formed, a 1:1 resonant pair is capable of migrating to small semimajor axes. In the case of 1:1 resonant migration, angular momentum and energy loss from the planets occurs at a rate that prevents a secular increase in eccentricity." (Laughlin et al., 2002). See their Fig. 10.

Detection:

Detection techniques

Most relevant papers on the detection techniques and detectability of exotrojan bodies.

Radial velocity

Several methodologies have been proposed to detect exotrojan planets using the effect of its gravitational pull on both the planet and the star. Here are some of the methods that have been proposed so far:

  • Nauemberg et al. (2002): Stability and Eccentricity for Two Planets in a 1:1 Resonance, and Their Possible Occurrence in Extrasolar Planetary Systems.

    "For an exact 1 : 1 resonance, the Doppler shift variation in the emitted light would be the same as for stars that have only a single planetary companion. But it is more likely that in actual extrasolar planetary systems there are deviations from such a resonance, raising the interesting prospect that Lagrange’s solution can be identified by an analysis of the observations. The existence of another stable 1 : 1 resonance solution that would have a more unambiguous Doppler shift signature is also discussed."

  • Ford et al. (2006): Observational Constraints on Trojans of Transiting Extrasolar Planets.

    "We present a novel method of detecting Trojan companions to transiting extrasolar planets that involves comparing the midtime of eclipse with the time of the stellar reflex velocity null. We demonstrate that this method offers the potential to detect terrestrial-mass Trojans using existing ground-based observatories."

  • Leleu et al. (2015): Detectability of quasi-circular co-orbital planets. Application to the radial velocity technique.

    "We determine a criterion for the detectability of quasi-circular co-orbital planets and develop a demodulation method to bring out their signature from the ob- servational data. We show that the precision required to identify a pair of co-orbital planets depends only on the libration amplitude and on the planet’s mass ratio. We apply our method to synthetic radial velocity data, and show that for tadpole orbits we are able to determine the inclination of the system to the line of sight."

  • Giuppone et al. (2012): Origin and detectability of co-orbital planets from radial velocity data.

    "We analyse the possibilities of detection of hypothetical exoplanets in co-orbital motion from synthetic radial velocity (RV) signals, taking into account different types of stable planar configurations, orbital eccentricities and mass ratios."

Transit signal

If the trojan is sufficiently large (e.g., Earth size) and it is by chance transiting the star, we should be able to detect the transit signal with the photometric precision provided by the Kepler mission and the forthcoming CHEOPS, TESS, and PLATO missions. There are some of the attempts to find these objects in the Kepler sample of planet candidates:

  • Janson et al. (2013): A systematic search for trojan planets in the Kepler data.

    "Systematic search for extrasolar trojan companions to 2244 known Kepler Objects of Interest (KOIs), with epicyclic orbital characteristics similar to those of the Jovian trojan families. No convincing trojan candidates are found, despite a typical sensitivity down to Earth-size objects. This fact, however, cannot be used to stringently exclude the existence of trojans in this size range, since stable trojans need not necessarily share the same orbital plane as the planet, and thus may not transit"

  • Hippke et al. (2015): A statistical search for a population of Exo-Trojans in the Kepler dataset

    "We present an analysis by super-stacking ∼ 4×104 Kepler planets with a total of ∼ 9×105 transits, searching for an average trojan transit dip. Our result gives an upper limit to the average Trojan transiting area (per planet) corresponding to one body of radius < 460km at 2-sigma confidence"

Transit timing variations

If the trojan is sufficiently massive (e.g., Earth mass) and is librating around one of the Lagrangian points of the system, this motion will induce a reflex motion of the planet. If by chance the planet transits its star as seen from Earth, this reflex motion will be reflected in a periodic variation in the time of the transit. These are the most relevant papers exploring this detection technique and their main concluions:

  • Ford et al. (2007): Using Transit Timing Observations to Search for Trojans of Transiting Extrasolar Planets.

    "We examine the sensitivity of transit timing observations for detecting Trojan companions to transiting extrasolar planets. We demonstrate that this method offers the potential to detect terrestrial-mass Trojans using existing ground-based observatories.."

  • Haghighipour et al. (2013): Detection of Earth-mass and Super-Earth Trojan Planets Using Transit Timing Variation Method.

    "Results indicated that while in general, the amplitude of TTVs fall within the detectable range of timing precision obtained from the Kepler’s long-cadence data, the prospects of the detection of Trojan planets are higher for super-Earth Trojans in slightly eccentric orbits around transiting Jovian planets with masses smaller than Jupiter.[...] How such Trojan planets form is a matter of on-going research. In general, one can think of three scenarios; in-situ formation around L4 and L5 Lagrangian points of a short-period giant planet, in-situ formation around the host giant planet followed by the inward migration of the planet- Trojan system, and formation in the inner part of the protoplanetary disk followed by capture in a 1:1 MMR during the migration of a giant planet."

  • Vokrouhlicky & Nesvorny (2014): Transit Timing Variations for Planets Co-orbiting in the Horseshoe Regime.

    "Here we study transit timing variations (TTVs) produced by mutual gravitational interaction of planets in the horseshoe orbital architechture, with the goal to develop methods that can be used to recognize this case in observational data."